Simulation results and Conclusions

In order to emphasize the diversity order created by coding at the transmitter side, all computer simulations have been conducted with the number of receive antennas $n_r=1$. Figures 4 and 5 illustrate the word error rate performance of a space-time BICM for $n_t=2$ transmit antennas, $n_c=2$ channel states, $s=2$ time period spreading and a coding rate $R_c=1/2$. Figure 4 illustrates the case with $n_t=4$ transmit antennas and a precoding spread factor $s=2$. At the first iteration, for $n_t=2$, IOM precoding slightly outperforms other rotations. After 10 detection/decoding iterations, IOM is outperformed by G-IOM and other algebraic rotations. The slight difference in performance is still apparent for $n_t=4$.

Figure 4: Word error rate of ST-BICM at iterations 1 and 10. MIMO channel $n_t=s=n_c=2$, $n_r=1$. Interleaver size $N=2048$ bits, rate 1/2 16-state $(23,35)$ convolutional code. QPSK modulation.

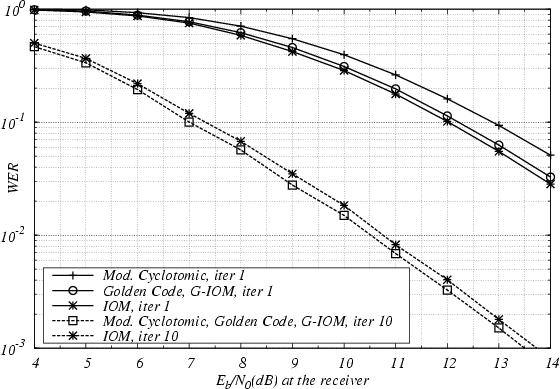Figure 5: Word error rate of ST-BICM versus outage limit. MIMO channel $n_t=s=n_c=2$, $n_r=1$. Interleaver size $N=2048$ bits, rate 1/2 16-state $(23,35)$ convolutional code. QPSK modulation.

Figure 6: Word error rate of ST-BICM at iterations 1 and 10 versus outage limit with gaussian input. MIMO channel $n_t=4$, $s=n_c=2$, $n_r=1$. Interleaver size $N=2048$ bits, rate 1/2 16-state $(23,35)$ convolutional code. QPSK modulation.

From the observed performance and the flexibility of linear precoders, we conclude the following. Cyclotomic rotations satisfying genie/DNA conditions are the best choice for precoding in space-time bit-interleaved coded modulations. These rotations are optimal in both algebraic and information theoretical senses. They exist for any set of MIMO channel parameters, mainly the number of transmit antennas and the precoder time-spreading factor.